How Many in a Legion?

The Book of Mark recounts a story of Jesus meeting, in “the country of the Gaderenes”, a man said to be possessed by a demon. When Jesus asks the name of the demon, the answer comes: “My name is Legion; for we are many.” Let’s focus on that word legion, because this is the earliest recorded use of the word to simply mean a large number.

Roman legionaries marching, from the Column of Marcus Aurelius, Rome, Italy, 2nd century AD. Picture Credit Barosaurus Lentus

The word “legion” used here is a reference to the Roman military unit, the mainstay of the Roman army through the years of the Republic and the Empire. But how big is (or was) a legion? Well, as you might have guessed, it’s complicated.

At first, the Roman legion was the entire Roman army. The name comes from a root word meaning “picked”: we might say “drafted”, it’s everyone called up for military service. The sixth Roman king Servius Tullius, who ruled in the 6th century BCE, is said to have been the first to call for a regular census of the people. This census created the administrative infrastructure which allowed the raising of an army, the legion, in the numbers required. From the start, an important sub-unit of the legion, was the century, nominally 100 soldiers, in fact usually a somewhat smaller number. The officer in charge of a century was of course a centurion.

When the Romans rid themselves of kings, the governance of the Republic came under the authority of two consuls, each of whom had charge of an army, a legion. In times of need, additional legions could be called up. In the fourth century BCE, two further permanent legions were established so that each consul now had charge of two. The core troops of the legion were the heavy infantry, who were divided into three lines. The front line, the hastati, were the least experienced, and were organised into 10 maniples of 2 centuries each containing 60 soldiers, for a total of 1200. The same number of principes, experienced soldiers, made up the next line. And finally, a line of veterans, triarii, only to be used if needed, were organised as 10 maniples of 2 centuries of 30 soldiers each. So taking account of 1200 hastati + 1200 principes + 600 triarii makes around 3000 heavy infantry (plus, of course, their 60 centurions).

Light infantry, used as skirmishers, were not as formally ordered, and might have numbered 1200, while around 300 cavalry officers would add to the strength, to bring the overall legion total to around 4500. But things don’t always stay the same.

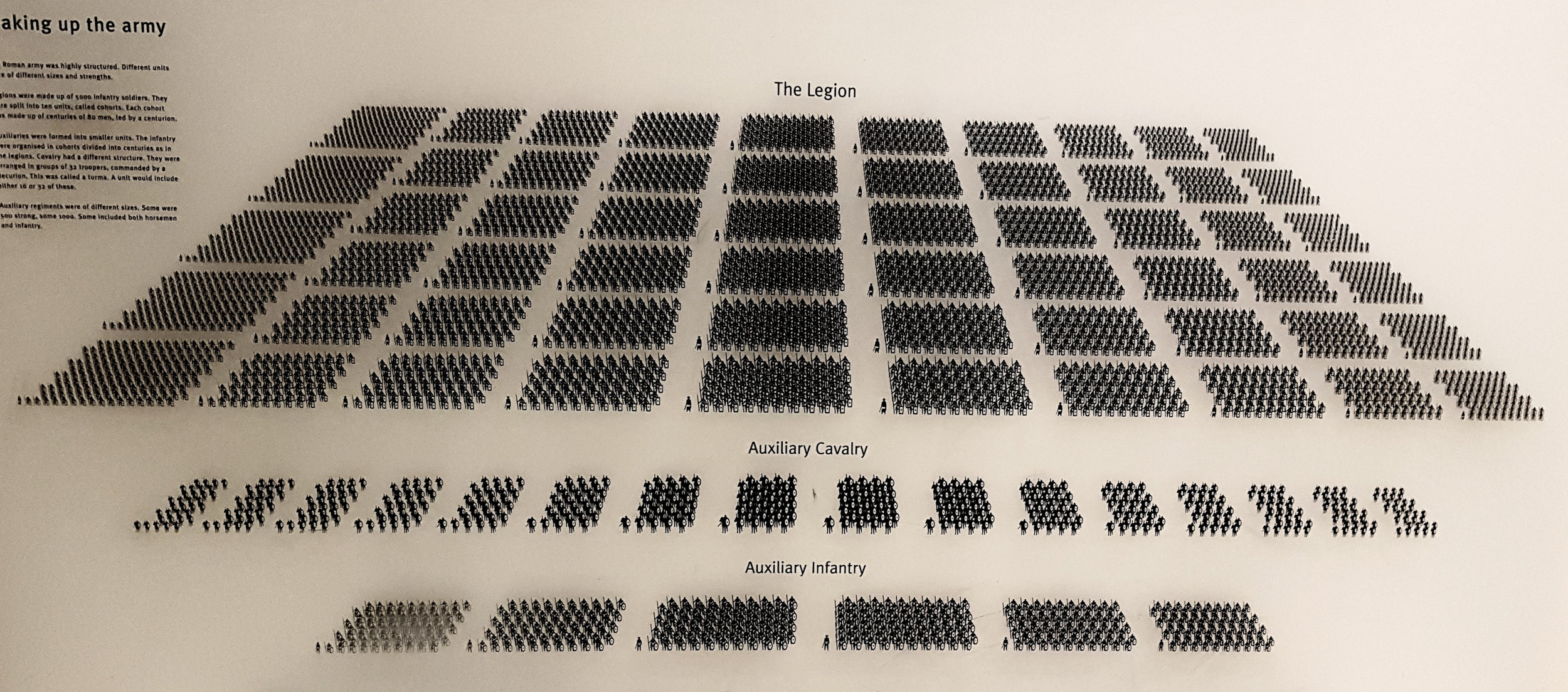
Display panel in the National Museum of Scotland.

The display panel shown above from the National Museum of Scotland (yes, there were Roman Legions in Scotland in the 1st and 2nd century CE) gives an excellent visualisation of the size of a Roman Legion, from a later period. The general Gaius Marius had reformed the organisation of the legion by around 100 BCE, and this display reflects his changes. The square blocks making up the bulk of the legion are the centuries, here shown 80-strong, and arranged into nine cohorts of six centuries each. The first (prima) cohort was exceptional, having only 5 centuries, but each twice as large as those in the remaining cohorts. By my reckoning the display shows a legion totalling 6193 men, with 528 horse.

So how big was a legion? More or less, somewhere between 3000 and 6000 strong. If we want a memorable number, a landmark number, 5000 will do.

Around the world in ... how long?

In around 200 BCE Eratosthenes, a Greek mathematician, geographer and librarian in Alexandria, calculated the size of the Earth.

He knew that in Syene, about 20 days' travel away, at midday on the summer solstice, the sun would be directly overhead. A shaft of sunlight would shine straight down a well shaft, and vertical columns would cast no shadows.

At the same time, in Alexandria, he measured the angle between the sun and the zenith. The angle he measured came to around 1/50 of a circle. He knew Syene to be, in his terms, 5000 stadia away. (A stadion was 125 paces, defined variously as between  157 and 185 metres. We now measure the Alexandria-to-Syene distance as 840 km, equivalent to a stadion that measures 168 m).  

Using a little geometry (which, after all, means "Earth-measuring"), he was able to calculate the circumference of the Earth. Depending on which definition of stadion you think he used, his answer (250,000 stadia) comes out to between 38,250 km and 46,250. Taking 50 times the distance from Alexandria to Syene (now modern-day Aswan) gives a result of 42,000 km. The true pole-to-pole circumference of the Earth is very close to 40,000 km. Eratosthenes pretty much nailed it. Remarkable for 2200 years ago.

---

If the distance from Alexandria was a 20-day journey on foot, then Eratosthenes could have worked out that that to walk all the way around the world (assuming such a route was possible) would have taken 1000 days. Using our modern measurement, that means 40 km a day, quite reasonable for a fit adult over good terrain.

As the title suggests, in Jules Verne's novel Around the World in 80 Days, his hero Phileas Fogg manages his circumnavigation in 80 days. In fact, as the map below shows, virtually all of the journey occurs north of the equator. The most southerly point is near Singapore, which is pretty much on the equator. The indirect route lengthens the journey. The fact that the circumnavigation is not at the Earth's widest latitude shortens it. These factors more or less balance out and the journey as mapped comes to approximately 38,000 km, just 5% less than the circumference of a great circle. Managing this in 80 days meant that Fogg made an average speed of 475 km per day.

Now, Phileas Fogg (and don't forget his faithful companion Passepartout) made it in 80 days, but how long would it take to circle the Earth using other means of transport? Here's a table showing how long it would take to travel 40,000 km in different ways:

To travel around the world	at avg speed	for	would take
On foot	5 km/h	8 h / day	1000 days
By car	100 km/h	8 h / day	50 days
By commercial plane	800 km/h	20 h / day	2 1/2 days
By military plane (B52)	940 km/h	continuous	42h 23 m
International Space Station	26,000 km/h	continuous	92 m

But to close, let's turn to Shakespeare. In A Midsummer Night's Dream, he has Puck say: I'll put a girdle round about the earth In forty minutes. That means Puck would be traveling at 1,000 km each minute: that's a megametre per minute.

A Trip to the Theatre

The Greek Theatre in the town of Taormina in Sicily has a spectacular view of the bay and of Europe’s highest volcano, Etna. The theatre itself dates to the third century BCE and although it's called the “Greek” Theatre, is largely the work of the Romans (the giveaway is that it is predominantly brick-built).

It’s regularly used as a concert venue, and the descriptive material suggests that it originally had a capacity of 5000. Is this claim credible? Let’s see if we can make an independent estimate of our own to compare.

As the image shows, the seating is arranged in sections, seven of them in total. So, trying to arrive at our own estimate of the capacity, we can tackle the simpler task of forming an idea of the capacity of one of those sections (and then later multiply by seven). Let’s take a closer look at one of the sections:

Counting the rows of seats (the lower ones are original stone, the upper ones are wooden bleachers), we get to 26 rows of seats currently in place. But there is some evidence of further, unrestored structure lower down, so we can guess that there might have been a further block of perhaps 12 rows there, for a total of 38 rows.

How many people might sit on one of those rows? More on the back rows, fewer in the front, but a reasonable figure for one of the middle rows might be 15 people.

So, we have 7 sections of 38 rows, each accommodating (on average) 15 people. Multiply those together to get 3990. It’s the right order of magnitude, but somewhat short of the 5000 claimed: perhaps that’s an optimistic claim?

But hold on! Those seven sections don’t make a complete semicircle. There is, in fact, on each side, space for a further section which would bring the total to nine sections, and that would make the total number of seats 5130. We can probably conclude that 5000 is a fair estimate.

Beware the Giant Ants! Or not ...

In the 1956 horror movie, Them, the plot revolves around "atomic testing in 1945 [that] developed ... dangerous mutant ants". 

The relationship between length, area and volume is sometimes called the "square-cube law": as the linear dimension of an object increases, so the surface area increases by the square of the multiplier, and the volume increases by the cube of the multiplier. The square-cube law explains why this great cliché of horror movies is so improbable. 

From the movie poster these ants look to be easily four metres in length, which means they must be around 1000 times longer than the 4 mm ants we are familiar with.But, in accordance with the square-cube law, a thousand-fold increase in length would mean a million-fold increase in measures of area and a billion-fold increase in measures of volume. Since the strength of the ants' legs would relate to the area of the cross-section of their limbs, while the mass of their bodies would relate to their volume, it follows that the ants' bodies would now be 1000 times too heavy for their limbs, and they would simply collapse under their own weight. The same would apply to the mass of their internal organs, now 1000 times too heavy to be contained by their chitinous "skins". Visualisation is left as an exercise to the reader.

How Many Tennis Balls Does It Take To Fill St Paul's?

There's a report available on the internet on the acoustic characteristics of St Paul’s Cathedral in London, and it has this little snippet of information: the interior volume of the cathedral is 152,000 m³. Is that a credible number? Let’s use a little bit of solid geometry to do some rough-and-ready cross-comparison.

A quick google at some pictures and measurements tells me that to a very rough approximation, the interior main body of St Paul’s can be approximated by a cuboid, roughly 50m wide, 150m long and 30m high. The famous interior Whispering Gallery is at 30 height and the exterior Stone Gallery around the dome is at 53 metres.

Based on this I don’t think it’s too unreasonable to imagine a simplified shape with the following interior dimensions (if you pushed all the interior stonework to the edges). Width 40m x height 25m x length of 140m, giving a total of 140,000 m³. The dome is around 30m in diameter and together with the cylindrical drum it sits on adds another approximately 30m to the interior vertical height. Working this through gives about another 18,000 m³ for the dome. We’ve reached a total of 158,000 m³ which is enough to convince me that the figure that the acoustic engineers used is probably close enough.

Now for the tennis balls. If you tumble a load of balls into a container, they won’t completely fill the space. If you pack them super-carefully you can bring the proportion of space filled to around 78%, but if you just let them settle for themselves, you can expect around 65% of the space to be filled. A tennis ball of 6.8cm diameter will have a volume of around 165 cm³, but when loosely packed, will occupy a volume of around 250 cm³, roughly a cupful. This means a box with a volume of one cubic metre will hold around 4000 tennis balls (not allowing for the “edge effect” which stops them from being so closely packed around the edges). And that means that the interior of St Paul’s will hold 152,000 times as much for a total of 608 million tennis balls.

But what if, instead of tennis balls, we used pool balls? With a diameter of 5.715 cm, their volume is just about 60% of the volume of a tennis ball. You can see where I’m going with this, can’t you? A metre cubed can accommodate 6700 pool balls, and if we multiply up, we get to 1,018,400 pool balls to fill St Paul’s. And that’s one way to visualise a billion.